Mathematics calculations are used in anatomy and physiology to provide additional insight into the information provided by the measurement of physiological quantities. The following exercises use a range of mathematical formulae that model various anatomic and physiological processes. The exercises also provide you with the opportunity to implement your general knowledge of rates, ratio and proportion.
While the calculations themselves are not intended to be arithmetically difficult, the examples are presented within a health science context and involve specialist terminology relating to human anatomy and physiology.
Image: http://www.hamilton.k12.nj.us/webpages/phearney/imageGallery/anatomy-physiology-student-tutorial-800x800.jpg
Examples
1. Rate of urine formation
QU: Given that 250ml of urine was produced in 30 minutes. What is the rate of urine formation (in ml/minute)?
Ans: The stated rate of 250 ml/30min needs to be converted into a rate per minute
= 250 ÷ 30 ml/min = 8.3ml/min
2. Respiratory rate
QU: If the number of inhalations in 20 seconds was 5, what is the respiratory rate (in breaths/min)?
Ans: The given rate of 5 breaths per 20sec must be converted into a rate per minute.
Generally the breaths are counted for 20 or for 30 seconds so that they may be converted into breaths per minute (60sec) easily (by multiplying by 3 or 2 respectively).
(5 breaths / 20 secs ) × 3 = 15 breaths / 60sec = 15 bpm
3. Heart rate
QU: If the radial pulse is counted for 15 seconds at 14 beats, what is the heart rate (in beats per minute)?
Ans: the given rate of 14beats/15sec must be converted into a rate per minute
= (14 beats / 15s) × 4 = 56beats/60s = 56bpm
4. Mean arterial pressure
Mean arterial pressure (MAP) is the "average" of the maximum blood pressure in the aorta (i.e., during systole) and the minimum blood pressure in the aorta (i.e., during diastole). Arterial blood pressure is written with larger number (systolic pressure) first, separated from the lower value (diastolic pressure) by a slash: eg 120/40 in units of millimetres of mercury.
MAP = diastolic pressure + ⅓ × (systolic pressure – diastolic pressure)
QU: what is the MAP for a blood pressure reading of 124/78? (systolic/diastolic)?
Ans: MAP = 78 + ⅓ ×(124 -78) = 78 + ⅓ ×(46) = 78 + 15.3 = 93.3mmHg
5. Total magnification for a microscope
Microscopes have two sets of lenses: the eyepiece lens (which you look through) and the objective lens (which is placed just above the specimen being viewed).
The eyepiece lenses have a magnification of 10X.
The 4 objective lenses have magnifications of 4X, 10X, 40X and 100X
Total magnification = eyepiece lens magnification × objective lens magnification
QU: What is the total magnification of a microscope with eyepiece lens of 10X and objective lens of 40X?
Ans: 10×40 = 400X magnification
Examples
6. Normal saline
A solution that is infused intravenously is "normal saline". It has a concentration of dissolved particles that is the same as the concentration of dissolved particles in blood. However saline contains only sodium chloride (Na+Cl- = table salt) dissolved in water. One kilogram of normal saline solution contains 9g of sodium chloride.
%conc = mass of solute ÷ mass of solution × 100
QU: what is the percentage concentration of sodium chloride in this solution?
Ans: %conc = 9g ÷ 1000g × 100 = 0.009 × 100 = 0.9%
7. Waist to hip ratio (WHR)
In many cases persons with extra fat located around the middle ('apple' shaped) are at higher risk for diseases such as heart disease and diabetes than those who carry weight around their hips and thighs ('pear' shaped). (A waist circumference >88cm for women or >102cm for men defines abdominal obesity).
WHR = waist circumference (cm) ÷ hip circumference (cm)
(the waist circumference = circumference at or just above the umbilicus while breathing out gently)
(the hip circumference = circumference at the widest point ie at greater trochanters which are the bony bits at the side of your hips)
QU: determine your WHR using the measured circumference of your waist and your hips
8. Ankle/Brachial index (ABI)
Ankle/Brachial index (ABI) is a non-invasive test used for assessing the arterial circulation in the lower limbs. Normally the blood systolic pressure measured in the feet is greater than or equal to the blood systolic pressure measured at the wrist.
ABI = systolic pressure at radial artery ÷ systolic pressure in posterior tibial artery
QU: Determine the ABI for a male when:
(use typical male values:
Brachial artery systolic pressure=119 mmHg;
Tibial artery systolic pressure (when supine) = 148 mmHg;
Tibial artery systolic pressure (when standing) = 216 mmHg)
Ans:
9. Body Mass Index (BMI)
Body mass index (BMI) combines measurements of height and weight to determine whether an individual is the appropriate weight for their height.
QU: Determine the Body Mass index (corrected to 1 decimal place) using your own height and weight. Classify yourself as underweight, of healthy weight, overweight or obese.
Ans: (for
example)
6. Mosteller formula for total body surface area
Pharmacology application: anti-cancer drugs are sometimes administered at a dose depending on the individual's body surface area (BSA), e.g. 200 mg/m2 (but the practice has been criticised as unscientific). BSA can be calculated from your measured height and mass.
QU: Use the Mosteller formula below to calculate body surface area (to 2 decimal places) for a person who is 176cm tall and has a mass of 72 kg:
[hint: Multiply height by weight, then divide the answer by 3600. Now use a calculator take the "square root" of this answer.]
Ans:
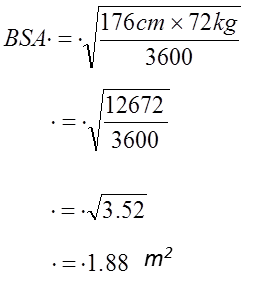
Examples
11. Percentage of body surface using "rule of 9s"
Total body surface area (TBSA) is an assessment of injury to or disease of the skin, such as burns or psoriasis. You can estimate the body surface area on an adult that has been burned by using multiples of 9, as follows:
Head = 9% of skin surface
Chest (front) = 9% (back = 9%)
Abdomen (front) = 9% (buttocks & back 9%)
Upper/mid/low back and buttocks = 18%
Each arm = 9% (front = 4.5%, back = 4.5%)
Groin = 1%
Each leg = 18% total (front = 9%, back = 9%)
QU: What percentage of the skin surface has been burned if both legs, the groin and the front chest and abdomen were burned?
Ans: both legs (18% x 2 = 36%), the groin (1%) and the front chest and abdomen (18%) were burned, hence 36 + 1 + 18 = 55% of the body surface.
12. ECG calculations
An ECG measures the variation with time of the voltage produced by the heart muscle as it contracts (see figure below). The graph is printed on moving graph paper marked in millimetres.
Since the paper speed was 25mm/second, each millimetre (mm) of paper corresponds to a time interval of 0.04 seconds.
Example: If an interval requires 4mm of paper its duration will be:
4mm × 0.04 sec/mm = 0.l6 seconds.
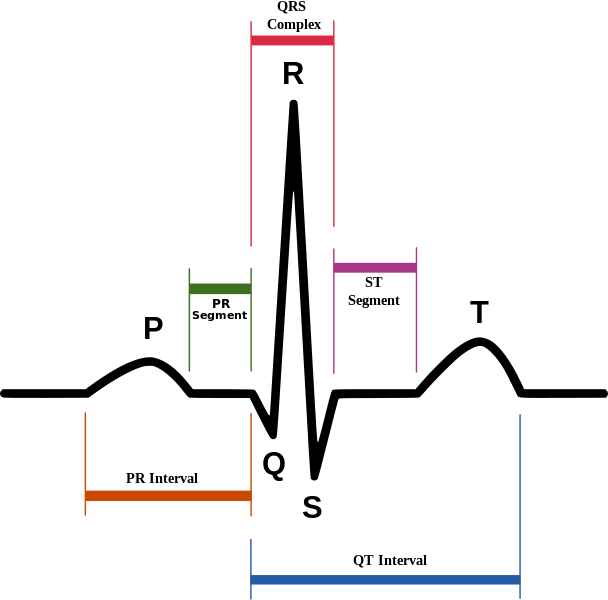
Image: http://www.dentalarticles.com/images/ecg1.png
QU: Calculate the time duration of:
25mm
5mm
2.5mm
Ans:
25mm × 0.04 sec/mm = 1.0 seconds = 1000ms
5mm × 0.04 sec/mm = 0.2 seconds = 200ms;
2.5mm × 0.04 sec/mm = 0.1s = 100ms
On the ECG graph, the time from the start of the "P wave" to the start of the "R wave" is called the P-R interval (it is normally less than 0.2 second)
QU: calculate the P-R interval (in seconds) if there is 4mm between the two waves.
Ans: P-R interval duration: 4mm × 0.04 sec/mm = 0.16s
The QT interval of the ECG graph varies with heart rate - becoming shorter at faster rates. It is usually corrected (to QTc) by using Bazett's formula:
QTc = QT ÷ √(RR)
QU: determine QTc given that QT = 12mm ; RR = 32mm (and convert mm to time using 1mm = 0.04s)
Ans: 12mm corresponds to 12×0.04 = 0.48s
13. GFR (creatinine clearance) in ml/min estimated with Cockroft-Gault formula
Creatinine clearance rate (CCR) is the volume of blood plasma that is cleared of creatinine per unit time and is a useful measure of how well the kidneys are working (renal function). Glomerular filtration rate (GFR) describes the flow rate of filtered fluid through the kidney. CCR is used for approximating the GFR in order to indicate of the health state of the kidney. (CCR of between 90 and 150 ml/min is healthy)
Creatinine clearance= [(140 - Age) x weight (kg) x F] ÷ (Plasma Creatinine × 0.8136) ml/min
(Where F = 1 if male, and 0.85 if female, Requires creatinine measurement in umol/L)
QU: Use plasma creatinine of 110 umol/L to determine creatinine clearance for a 59yo male of mass 82kg.
QU: Use plasma creatinine of 80 umol/L to determine creatinine clearance for a 36yo female whose weight is 72kg.
Ans (for male): CCR = [(140 – 59)×82×1] ÷ (110×0.8136) ml/min
[(81)×82] ÷ 89.5
= 6642 ÷ 89.5
= 74.2 ml/min
Ans (for female): CCR = [(140 – 36)×72×0.85] ÷ (80×0.8136) ml/min
= 6365 ÷ 98.3
= 64.8 ml/min
14. Lung volumes
Forced vital capacity (FVC) is the maximum volume of that can be forcefully exhaled from the lungs after a maximum inhalation. Forced expiratory volume in one second (FEV1) is the volume of air that can be forcefully exhaled (with a maximum effort) in 1s. FEV1 should be greater than 80% of FVC.
QU: given an FVC of 4.93L and an FEV1 of 4.24 L, what is FEV1%?
Ans: FEV1% = FEV1 ÷ FVC × 100% = 4.24 ÷ 4.93 × 100% = 86%
15. Lung residual volume calculation
Even when you are fully exhaled, your lungs contain some air. This air volume is called the "residual volume" (RV) and is difficult to measure. However, an estimate of RV can be made using the following formula:
For males: RV = (2.29 × height) – (0.012 × weight) - 1.72 litres
For females: RV = (2.63 × height) – (0.02 × weight) – 1.78 litres
(Note: height = height in metres, e.g. 1.76 m; weight = weight in kg, e.g. 72 kg)
QU: Estimate your lung RV by substituting your height (in m) and your weight (in kg) into the formula.
Ans: if a male ht = 176cm & weight =72kg:
RV = (2.29 × 1.76) – (0.012 × 72) - 1.72 litres
= 4.03 – 0.864 – 1.72 L
= 1.45 L
Examples
16. Oxygen transport and haemoglobin
Oxygen is transported around your body by blood while attached to the protein called "haemoglobin" (Hb). Hb is located inside your red blood cells (rbc) of which you have millions. We will calculate how many you have.
(Given that 1 microlitre (1mm3 ) of blood contains about 5 million (5 ×106 ) rbc, each rbc contains about 280 million (280 ×106 ) haemoglobin (Hb) molecules)
QU: How many molecules of haemoglobin does the 5.5 litres of blood within an average human contain?
[hint: first calculate the number of microliters of blood in 5.5L. ]
Ans: Humans have about 5.5 litres of blood and 280 ×106 Hb molecules per rbc.
5.5 litres = 5500 millilitres
= 5 500 000 microlitres
= 5.5 × 106 microlitres
(5.5 × 106 ul) × (5 ×106 rbc per ul) = 27.5 × 1012 rbc per human
(280 ×106 ) Hb molec/rbc × (27.5 × 1012 ) rbc/human = 7700 × 1018
= 7.7 × 1021 Hb molecules/human
(= 7 700 000 000 000 000 000 000 molecules of Hb/human)
17. Anion Gap
Anion Gap (IONGP) is a characteristic of blood electrolytes that is routinely determined for diagnostic purposes. It is the difference between measured concentration of cations (positive ions) and anions (negative ions) in the serum. (healthy range = 7-17 mmol/L)
Anion Gap = (sodium + potassium) – (chloride + bicarbonate)
= (Na+ + K+) – (Cl- + HCO3-)
QU: Calculate the anion gap for a patient whose electrolytes concentrations (in mmol/L) are: sodium=140, potassium=3.9, chloride=101, bicarbonate=26. Is it in the normal range ?
Ans:
= (140 + 3.9) – (101 + 26)
= (143.9) – (127)
= 16.9 (within normal range)
18. Blood pH
The following calculation involves logarithms. You may wish to work through the module on Logarithms first.
Blood pH is a way of expressing the concentration of hydronium (that is hydrogen) ions in the blood which gives us a measure of its acidity. Blood pH is found by calculating the negative logarithm of the hydrogen ion concentration. Blood pH is strictly regulated by the body to be between 7.35 and 7.45 in healthy people.
pH = -log10(H+ conc in mol/L)
QU: Use your calculator's logarithm function to calculate blood's pH given that its H+ concentration is 4.0 × 10-8 mol/L
Ans:
pH = -log(4.0 × 10-8)
= -(log (4.0) + log (10-8))
= -(0.6026 -8)
= -(-7.4)
= 7.4
Calculator example: enter:
10, xy,
8, +/-,
×, 4, =, (4 × 10-8)
Log, =, (-7.4)
So log(4 × 10-8) = -7.4